Pascal's Triangle

Beauty in Mathematics
Fibonacci sequence
Let us consider light switches

- How many ways can 0 switches be set?
Question may seem weird, but mathematicians want to be
thorough!
We could say 0 or 1, but 1 works better!
So zero switches have one state.
- How many ways can 1 switch be set on?
One, it is on!
- How many ways can 1 switch be set off?
One, it is off!
- How many ways can 2 switches be set all on?
One, they are all on!
- How many ways can 2 switches be set all off?
One, they are all off!
- How many ways can 2 switches be set one on, one off?
Two ways, switch one is on, switch two is off,
or switch one is off, switch two is on.
- How many ways can 3 switches be set all on?
One, they are all on!
- How many ways can 3 switches be set all off?
One, they are all off!
- How many ways can 3 switches have 1 switch on (= 2 off)?
3 ways: switch 1 is on, switch 2 is on, or switch 3 is on.
- How many ways can 3 switches have 2 switches on (= 1 off)?
3 ways: switch 1 is off, switch 2 is off, or switch 3 is
off.
Relationship of different rows
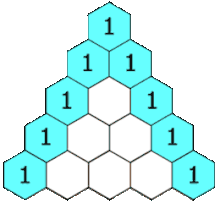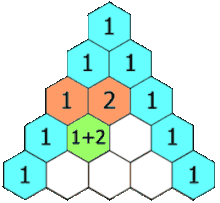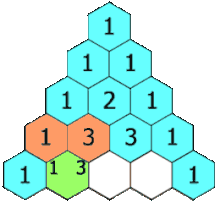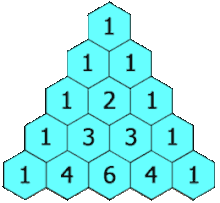
Why does this relationship hold? Let's go back to the light switches.
Set one switch on or off. Then there are:
- You chose ON: (n - 1) choose (k - 1)
- You chose OFF: (n - 1) choose k
ways left to choose the rest.
Relationship to binomials
(a + b) = a + b
(a + b)2 = a2 +2ab + b2
(a + b)3
= a3
+3a2b
+ 3ab2
+ b3
(a + b)4
= a4
+4a3b
+6a2b2
+ 4ab3
+ 3b4

Relationship to the Fibonacci Sequence
Amazingly, the diagonals of the Pascal's Triangle sum to the members of
the Fibonacci sequence!
External Links

Blaise
Pascal




